Models of Surfaces
Humans have been constructing approximations of polyhedra and simple geometric solids for a long time. Spherical forms that show all five regular polyhedra have been found in Scotland dating as far back as 2000 BCE [2]. However, models of more complicated three dimensional surfaces do not appear until the beginning of the 19th century after more sophisticated mathematical theories led mathematicians to attempt to visualize such forms.
In the 1700’s, Gaspard Monge developed descriptive geometry, which involves representing three dimensional objects in two dimensions (for instance, by sketching a projection of the object). His ideas were developed at a school for military engineering and were a military secret until after the French Revolution. Monge made models of ruled surfaces for instructional purposes; his are the earliest known models of this type [1, 11].
Monge’s student, Thèodore Olivier, also built models. Olivier taught descriptive geometry at the École Centrale des Aries et Manufactures and the Conservatoire National des Arts et Métiers and designed models of ruled surfaces some of which were manufactured by the firm of Pixii, Père, and Sons. Their successor, Fabre de Lagrange, continued to manufacture the models [11], selling models to locations such as the South Kensington Museum in London (now known as the Science Museum) [14].
Around the mid-1800’s, the “golden age” of model building began. Many mathematicians began to build models out of a variety of materials, including plaster, cardboard, metal, and string. Many of the model-builders were German, and the names of those involved include influential mathematicians such as Eduard Kummer, Felix Klein, and Alexander Brill. Felix Klein was a major proponent of both visual intuition and model-building. After the 1893 World’s Columbian Exposition (World’s Fair) in Chicago, Klein gave a series of lectures at Northwestern University in which he said,
“I wish to insist in particular on what I regard as the principal
characteristic of the geometrical methods that I have discussed today:
these methods give us an actual mental image of the configuration
under discussion, and this I consider as most essential in all true
geometry”
The German exhibit at the Chicago World’s Fair featured a display of models which were available for purchase by universities [11].
In the fourth lecture at Northwestern, Klein discussed the shape of algebraic curves and surfaces, making reference to models that were displayed during the World’s Fair. One such model is diagonal cubic created by Alfred Clebsch (see Figure 1).
“In 1872 we considered, in Göttingen, the question as to the
shape of surfaces of the third order. As a particular case,
Clebsch at this time constructed his beautiful model of the
diagonal surface, with 27 real lines”
Any smooth surface which is the zero locus of a polynomial of degree three has exactly 27 straight lines lying on the surface. In the diagonal cubic all 27 of these lines are real. In the plaster model in Figure 1, these lines have been etched on the surface. In this model, several “passages” (as they were called in the classic literature) can be seen. Klein had the idea to generate all of the possible types of cubic surfaces by collapsing and deforming these passages (for some details, see [6]) and a series of models illustrating this was executed by Klein and his student Carl Rodenburg. For example, collapsing three of the passages results in a surface with three ordinary double points which contains nine lines (see Figure 1).
On left: Clebsch diagonal cubic, showing the 27 real lines lying on the surface. Brill Series 7, Number 1. On right: Cubic with three A1 double points. Brill Series 7, Number 8. From the MIT collection.
There are many other gems to be found in a collection of mathematical models, including the hyperboloid of one sheet and the helicoid (see Figure 2) as well as Kuen’s Surface and the generalized helicoid of constant negative curvature (see Figure 3).
Many of the models built were reproduced and sold by publishing houses (especially the German publishing house of Ludwig Brill, which was later taken over by Martin Schilling) to schools and museums around the world. In 1911, the catalogue of Schilling models contained 40 series, which included 4000 models and devices [6]. By 1932, Martin Schilling informed the mathematics institute at Göttingen that
“in the last years, no new models appeared”
The most productive phase of model-building was over, perhaps due in part to the shift in the mathematical culture away from intuition and visualization and towards formality and rigor. Many of the models, however, survived in universities and museums, sitting in dusty cases when they were no longer brought out as part of mathematics instruction. There they awaited discovery by the non-mathematical world.
Birth of Modern Art
Until the late 19th century, western art was mostly representational. That is, art consisted of drawings, paintings, and sculptures that were intended to represent some aspect of the physical world — people, animals, landscapes, and common objects. In the years preceding the First World War, a new kind of artistic movement began. This was a movement of abstract art, or art that was not intended by be representational. Wassily Kandinsky is generally regarded as having been the first western artist to paint purely abstract pictures. This first wave of abstraction was widened by movements such as Dada, de Stijl, and Constructivism.
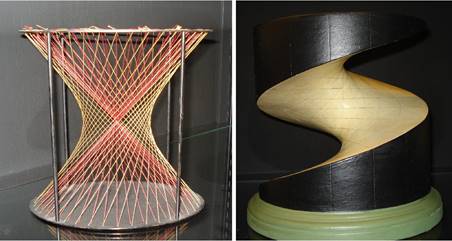
On left: Hyperboloid of one sheet with asymptotic cone. Unknown origin, but possibly Brill Series IV, Number 1. On right: Helicoid, showing the generating line. Brill Series 8, Number 6a. From the MIT collection.
On left: Two views of Kuen’s Surface. Brill Series 8, Number 1. On right: Generalized Helicoid. Brill Series 5, Number 4. Both of these surfaces have constant negative curvature. From the MIT collection.
Another kind of modern art was being developed during the time in between the world wars, the Surrealist movement. The Surrealists were not trying to remove representation from art (like Constructivists) nor to destroy traditional art (like Dada) but instead to give a voice to the unconscious and irrational.
Both the Surrealists and Constructivists seem to have had some exposure to the mathematical models of surfaces. These models of geometric surfaces have an eerie beauty; it is not surprising that artists would be intrigued by them. It is even less surprising when you put their interest in context. The mid- to late-nineteenth century was a turbulent time in mathematics. Old ideas, such as Euclidean geometry, were being turned on their heads. Many revolutionary mathematical ideas made their way into the public sphere and sparked the imagination of writers and artists alike. Writers such as H. G. Wells and artists like Marcel Duchamp were fascinated with non-Euclidean geometry and the idea of a spatial fourth dimension. The surrealists found that
“non-Euclidean geometry signified a new freedom from the tyranny
of established laws”
Culturally, mathematics represented both scientific progress and the potential for chaos.
Naum Gabo and the Constructivists
Constructivism was an artistic and architectural movement that began in Russia in the early 20th century. Naum Gabo and his brother Antoine Pevsner were, to a large extent, responsible for popularizing and spreading the movement outside of Russia, notably to Paris and England. Constructivists also had an influence on the Abstraction-Creation group, the de Stijl movement, and the Bauhaus.
It is likely that Gabo saw mathematical models on display while he was a student in Munich. Gabo had some interest in art as a teen, but went to the University of Munich to study medicine. He took other scientific coursework while he was there, particularly physics and engineering [13]. Notably, he also followed some courses at the Technical University in Munich, where there were certainly mathematical models on display, as Felix Klein and Alexander Brill produced and studied models in problem sessions with students [6]. In his 1936 Museum of Modern Art exhibition catalog Cubism and Abstract Art, Alfred Barr made even more of Gabo’s connection with models, stating that Gabo
“had been studying mathematics in Munich and had made mathematical
models”
This statement is probably inaccurate, but it does show that the topic of Gabo’s connection with mathematical models had surfaced during Barr’s research.
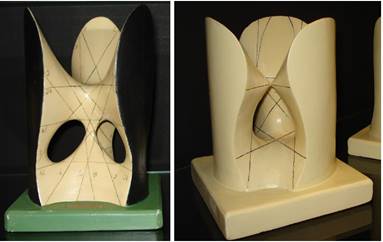
Gabo’s early cubist-influenced sculptures such has Head No. 2 (see Figure 4) are reminiscent of cardboard models of surfaces made with interlocking cross-sections, such as would have been on display at the university. Pictures of such models could also be found in encyclopedias of the time.
This early similarity of Gabo’s work to certain mathematical models may simply be coincidence, but later influence of models on Gabo’s work in the 1930’s is clear. In their book, Constructing Modernity: The Art and Career of Naum Gabo, Christina Lodder and Martin Hammer note that a 1936 drawing Study for Construction in Space: Crystal appears to be a tracing of a figure from the article “Mathematical Models” in the 14th edition of the Encyclopaedia Britannica [13]. Indeed, the Encyclopaedia drawing has many similarities to the final sculpture Construction in Space: Crystal (1937–9, cellulose acetate, Tate Modern, London).
Others sculptures and drawings show evidence of Gabo’s encounter with mathematical models. For instance, Gabo’s 1933 Sketch for a Stone Carving (see Figure 4) is reminiscent of a ruled surface or helicoid (see Figure 2) which would have been on display at the Poincaré Institute in Paris. Gabo was living in Paris at the time this sketch was done.

Works by Noam Gabo. On left: Constructed Head No. 2, 1916 (enlargement 1975), stainless steel, Nasher Sculpture Center, Dallas, Texas. On right: Sketch for a Stone Carving, 1933, crayon on paper, Gabo family collection.
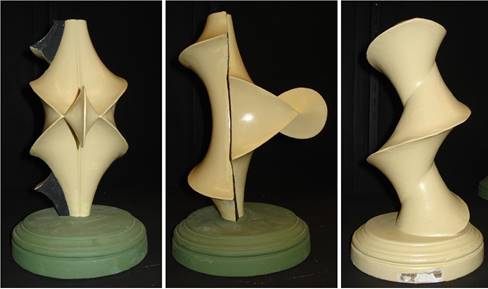
Antoine Pevsner was the brother of Naum Gabo. Constructions of Pevsner’s from the mid-1930’s show evidence of a possible influence of mathematical models. Pevsner began his artistic career as a painter, and during the 1920’s Gabo encouraged his brother to pursue sculpture and taught him constructive techniques. Pevsner always denied any direct influence of mathematics on his work. However, his Developable Surface series was perhaps inspired by models of ruled surfaces (see Figure 2). Mathematically, the term developable refers to a surface which can be constructed from a flat plane without stretching or tearing (at least locally). Such a surface can thus be swept out by lines precisely in the manner of Pevsner’s sculptures (see Figure 5).
British sculptor Barbara Hepworth had contact with Naum Gabo while Gabo was in England — from 1936 to 1946. Hepworth may have seen mathematical models before she encountered Gabo. In December 1935, Hepworth sent a letter in which she said that architect John Summerson had told her that there were
“some marvelous things in a mathematical school in Oxford —
sculptural working out of mathematical equations — hidden away
in a cupboard”
and that she intended to go and look at them soon [7]. of Hepworth’s work exhibits mathematical influence. The sculpture Helicoids in Sphere (1938, lignum vitae on original base, private collection), for instance, has similarities to a mathematical model of a surface known as Steiner’s Roman Surface. Other sculptures, such as Pelagos (1946, painted wood and strings, Tate Modem, London) and Spring (see Figure 5) echo mathematical models in their form and in their use of string. During the 1930’s, Hepworth was producing sculptures using string and plaster as in Sculpture with Colour (Deep Blue and Red) (1940, painted plaster and string, Tate Modem, London). Both of these materials were materials widely used in the mathematical models.
Henry Moore is another British sculptor who is sometimes associated with constructivism. Moore stated more than once that his use of string in his sculpture, which began in 1937, was influenced by seeing models at the Science Museum in London.
“I was fascinated by the mathematical models I saw there,
which had been made to illustrate the difference of the form
that is halfway between a square and a circle. One model had
a square at one end with 20 holes along each side… Through these
holes rings were threaded and lead to a circle with the same
number of holes at the other end. A plane interposed through
the middle shows the form that is halfway between a square and
a circle… It wasn’t the scientific study of these models but
the ability to look through the strings as with a bird cage
and see one form within the other which excited me”
The influence of such models can be seen in pieces such as Stringed Figure No. 1 (1937, cherry wood and string, Hirshhorn Museum and Sculpture Garden, Washington, DC).

On left: Antoine Pevsner, Construction in the Third and Fourth Dimension, 1962, Princeton University, courtyard of Jadwin Hall. On right: Barbara Hepworth, Spring, 1966, bronze with strings, on loan from Hepworth estate to the Barbara Hepworth Museum in St Ives.
Man Ray and the Surrealists
In The History of Surrealist Painting, Marcel Jean suggests that it may have been Max Ernst who brought the mathematical models into the surrealist consciousness.
“Max Ernst had originally come across these constructions in
the Institute Henri Poincaré and had mentioned them to the director
of Cahiers d’Art, Christian Zervos, who
in his turn had asked Man Ray to photograph them”
This is backed up by Neil Baldwin’s account in his book on Man Ray, in which he says this was a series
“of photographs of items created in the 1880’s by a physicist
attempting to render algebraic formulae correctly. Max Ernst had
taken Man Ray to see the objects on display at the Poincaré
institute in Paris and had photographed them in a deliberately
impressionistic style”
And in Man Ray’s own words in the film A Life in the Day of Man Ray he says that he “was told about some mathematical objects at the Institute Poincaré in Paris,” although he does not mention who told him.
Man Ray then executed a series of photographs, entitled Mathematical Objects, which were studies on models at the Poincaré Institute. Some of these photographs appeared in a 1936 issue of Cahiers d’Art along with an essay on mathematics and abstract art by Christian Zervos.
Man Ray’s photographs, as well as his later series of paintings based on them (see Figure 6), gave mathematical models a lot of exposure. The surrealists displayed mathematical objects in their May 1936 show “Exposition Surraliste d’ Objets” at the Galerie Charles Ratton in Paris. In his famous “Crisis of the Object,” André Breton writes,
“The laboratories of mathematical institutions throughout
the world already display side by side objects constructed according
to both Euclidean and non-Euclidean principles, equally mystifying
in appearance to the layman, but which nevertheless bear a fascinating
and equivocal relationship to each other in space as we generally
conceive it”

Shakespearean Equation: King Lear, 1948, oil on canvas, Hirshhorn Museum and Sculpture Garden, Washington, DC. This painting is based on a model published by Brill-Schilling of a Kummer Surface with 8 double points.
Man Ray’s interest in the mathematical objects also may have had an influence on the 1936 International Surrealist Exhibition at the New Burlington Galleries in London. This show was supported financially and organized by Roland Penrose, who was befriended by Man Ray and André Breton in Paris. Man Ray went back and forth between London and Paris, carrying art in preparation for the show [3]. His photographs of mathematical objects were on display at the show, which was open from June 11 through July 4, 1936, with attendance of 1,500 people per day. Even the catalogue from this exhibition reveals a fascination with mathematical models — the front cover of the catalog is a collage by Max Ernst featuring a statue with a reptilian head holding and standing near a number of mathematical models, including Kuen’s Surface (see Figure 3). Man Ray’s photographs showed up at yet another big exhibition of the time, the 1936–37 Fantastic Art, Dada, and Surrealism exhibition at New York’s Museum of Modern Art.
Other surrealists may have been inspired by seeing the models and their photographs. Max Ernst, who did the cover of the London exhibition catalog, did several other collages and paintings that seem to relate to mathematical models. Examples include The Feast of the Gods (1948, oil on canvas, Museum of the 20th Century, Vienna, Austria), Chemical Nuptials (1948), and Young Man Intrigued by the Flight of a Non-Euclidean Fly (1942–7) which contains several forms reminiscent of mathematical models such as the Spindle Cyclide and the Horn Cyclide.
Models and Art Today
The collections of models that inspired Gabo, Hepworth, and Man Ray can still be seen in universities and museums around the world. Here are a few of the many locations models can be found:
- Massachusetts Institute of Technology. MIT has a substantial collection of Brill-Shilling plaster models which are in very good condition. The models can be viewed in the corridors of the mathematics department, building 2.
- University of Arizona’s collection of models can be viewed online (http://math.arizona.edu/ models/). This collection includes a number of models by Richard Baker.
- University of Illinois Urbana-Champagne has a large collection of models in Altgeld Hall. This collection is unique because the majority of models were built specifically for the university by Arnold Emch, who was hired in 1911 to expand the university’s model collection.
- Smithsonian Institution. Over the years, a number of schools have given their collections of sometimes crumbling models to the Smithsonian. Peggy Kidwell (e-mail) curates the collection.
- Union College in Schenectady, NY, has a collection of Olivier models. These are currently housed in the library special collections but will eventually be moving back to the math department.
- The Poincaré Institute in Paris, where Man Ray went to photograph models, still has over 400 models. The collection is housed in the library.
- Dresden University of Technology in Germany has a collection of over 400 models, a number of which are viewable online at http://www.math.tu-dresden.de/modellsammlung/index.php.
These models are also still inspiring artists. In 2004, photographer Sugimoto Hiroshi did a series of photographs of plaster models from the collection of the University of Tokyo. The photographs have seen several exhibitions and were featured in the New York Times in 2005. You can see a few photographs online at http://www.sugimotohiroshi.com/conceptualforms.html and others can be found by searching the archives of the New York Times. Jonathan Chertok is an architect who has been recreating mathematical models from the Brill-Schilling catalog using rapid prototyping and other technologies. His work can be found at http://www.universaljointdesign.com/.